Complex Analysis: #12 Index of a Point with Respect to a Closed Path
for all t ∈ [t0, t1]
Definition 10
The number θ(t1) ∈ ℤ is called the index of the point z0 with respect to the closed path γ. Sometimes it is also called the winding number of γ with respect to z0. It is denoted νγ(z0).
Theorem 24
Let α and β be homotopic closed paths, homotopic by a homotopy H : Q → ℂ. Assume that z0 ∉ H(Q). then να(z0) = νβ(z0).
Proof
Let θα be the corresponding path in ℝ (corresponding to α) and let θβ be the path in ℝ corresponding to β. Then the homotopy from α to β induces a homotopy of the path θα to θβ in ℝ. Since the endpoints thus remain fixed, the index remains unchanged.
Theorem 25
Let γ, z0 and νγ(z0) be as in the definition (but this time we assume that γ is continuously differentiable). Then
Proof
Obviously the function 1/(z − z0) is analytic in ℂ \ {z0}. For simplicity, assume that z0 = 0 and γ(0) = γ(1) = 1 = ei·0 , where γ : [0, 1] → ℂ \ {0}. Write γ(t) = r(t)e2πiθ(t). Let
hτ(t) = e2πiθ(t)(t + (1 − t)r(t)),
for τ, t ∈ [0, 1]. This defines a homotopy from γ to a new path γ, which lies on the unit circle. But
Theorem 26 (Cauchy’s Theorem: Complicated version)
Let f : G → ℂ be an analytic function defined in a region G of ℂ. Let γ : [0, 1] → G be a continuous closed path in G such that νγ(a) = 0 for all a ∉ G. Then ∫αf(z)dz = 0.
Proof
Since the (image of the) path γ is a compact subset of G, there exists an ∈ > 0 such that for all t ∈ [0, 1], we have B(γ(t), ∈ ) ⊂ G. Let n ∈ ℕ be sufficiently large that 1/n < ∈ /√2. Then split up the whole complex plane ℂ into a system of small squares of the form
where p, q ∈ ℤ. γ meets only finitely many of these small squares, and each of the squares which γ does meet is completely contained in G. Our proof now consists in altering γ, one step after the next, through processes which can be achieved using a homotopy. In the end we get a version of γ which is so simple that the theorem becomes obvious.
- Let Q be one of the squares which γ meets. If γ only runs along the boundary of Q without entering it’s interior, then there is nothing further to do in this step of the proof. On the other hand, if γ does enter the interior of Q, then we perform a homotopy on it, moving it to the boundary of Q, but leaving all points of γ which are not in the interior of Q unchanged. Basically, we take an interior point of Q which is not a point of the path, then we push the part of γ which is in the interior of Q radially from that point out to the boundary. Do this with all the Q’s which are on the path, then finally, if necessary, move the endpoint γ(0) = γ(1) to a vertex of one of these squares. [Alternatively, and without loss of generality, we may assume that the endpoint of γ is already in a corner of the lattice] The end result of all this is a homotopy, moving γ so that at the end of the movement it is contained within the lattice of vertical and horizontal lines which make up the boundaries of all the small squares. For simplicity, let us again call this “simplified” version of the path γ.
- Now it may be that this simplified γ is still too complicated. Looking at each individual segment of the lattice, it may be that during a traverse of some particular segment, we see that γ moves back and forth in some irregular way. So again using a homotopy, we can replace γ with a new version which traverses each segment of the lattice in a simple linear path.
- We are still not completely happy with the version of γ which has been obtained, for it might be that γ has a winding number which is not zero with respect to one of the squares Q. That is, let q be an interior point of Q. What is νγ(q)? If it is not zero, then we can alter γ using a homotopy, changing the winding number with respect to this particular square to zero and not altering the winding number with respect to any of the other squares. The idea is simply to add a bit on to the end of γ, going out to a corner of the offending square along the lattice, then around the square a sufficient number of times to reduce the winding number to zero, returning to that corner, then returning back along the same path through the lattice to the starting point. Since γ is compact, there can be at most finitely many such offending squares. At the end of this operation, we have ensured that γ has winding number zero with respect to all points of ℂ which do not lie on the path γ.
Our curve is now sufficiently well simplified for our purposes. The reason for this is that for every segment of the lattice which γ traverses, it must be that it traverses the segment the same number of times in the one direction as it does in the other direction. To see this, let S be some segment of the lattice. To visualize the situation, imagine that it is a vertical segment. Assume that γ traverses the segment u times in the upwards direction, and d times in the downwards direction. Now S adjoins two squares, say Q1 on the left-hand side, and Q2 on the right-hand side. Let us now alter γ, moving the downwards moving parts which traverse S leftwards across Q1 to the other three sides of Q1. On the other hand, the upwards moving parts of γ are moved rightwards across Q2 to the other three sides of Q2. Let z1 be the middle point of the square Q1, and let z2 be the middle point of Q2. Before this movement, the winding number of γ with respect to both points was zero. However, afterwards, νγ(z1) = a and νγ(z2) = b. Yet they are in the same region of ℂ\ γ. Thus the points must have the same winding numbers and so a = b.
Finally, after all this fiddling, we see that we must have ∫γf(z)dz = 0 since the total of the contributions from the path integrals along each of the segments of the lattice adds up to zero in γ each case.
Up to now we have always been performing path integrals around single closed paths. This is quite sensible. But sometimes it is also convenient to imagine two or more closed paths. So let say γ1, . . . , γn be n paths, each of which are piecewise continuously differentiable and closed in some region G. We can think of them together, and call them a cycle, denoted by the letter Ω say. [More generally, we might allow paths which are not necessarily closed. In this case one speaks of “chains”, but we will not persue this idea further in this here.] Then if f : G → ℂ is a function, we might be able to perform the path integrals over each of the γj separately, and thus we can define the integral over the whole cycle to be simply the sum of the separate integrals.
Furthermore, if Ω is a cycle and z0 is a point not on any path of the cycle, then we can define the index of z0 with respect to the cycle to be the sum of the indices of z0 with respect to the individual closed paths in the cycle. On the other hand, if we want, we can connect the endpoints of the paths in a cycle together to make a single larger closed path, thus showing that theorem 26 is also true for cycles.
Theorem 27
Again, let G ⊂ ℂ be a region, f : G → ℂ be analytic, γ a closed curve in G with winding number zero with respect to all points of ℂ not in G. Let z0 ∈ G be a point which does not lie on the path γ, then
Proof
This is a straight-forward generalization of theorem 6. For r > 0 sufficiently small, we have
Let β(t) = z0 + r · e2πit be the path we are thinking about in this path-integral. (Here t ∈ [0, 1].) Obviously we have νβ(z0) = 1. Now take the cycle consisting of the given path γ, together with −νγ(z0) copies of the path β. (If this is a negative number, then we should travel around β in the reverse direction.) We assume that r is so small that the closed disc D(z0, r) is contained in G and furthermore the path γ does not meet D(z0, r). Then z0 has index zero with respect to the cycle consisting of γ − νγ(z0)β. Thus, according to theorem 26,




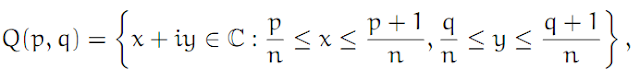




No comments:
Post a Comment
If it's a past exam question, do not include links to the paper. Only the reference.
Comments will only be published after moderation