• Physics 9702 Doubts | Help Page 77

Question 410: [Waves]
A point source of sound emits energy equally in all directions at a constant rate and a person 8m from the source listens. After a while, the intensity of the source is halved. If the person wishes the sound to seem as loud as before, how far should he be from the source?
A 2 m              B 2√2 m                      C 4 m              D 4√2 m                      E 8√2 m

Reference: Past Exam Paper – J84 / II / 11



Solution 410:
Answer: D.
The loudness of sound is measured by the intensity.

{Energy spreads out uniformly in 3-dimensional space. Hence, the power is spread across the area of a sphere.}
The intensity of sound (at a point) follows the inverse square law, that is, the intensity is inversely proportional to the square of the distance apart.

Intensity, I = (Power P of the source) / 4πr² where r is the separation

Initially, the person is at a distance r = 8m from the sound source.
Intensity (at a distance of 8m), I = P / 4π(8)² = P / 256π

Since the intensity is now halved (at all points), the power (of the source) should have also been halved. But for the same loudness of the sound at a new distance r_n, the intensity at the new position of the listener must be the same as before (at a distance of 8m). That is,
New intensity I = 0.5P / 4πr_n²  
(this intensity is the same as initially at a distance of 8m, but the power of the source is now halved)
Initial intensity I = P / 4π(8)² = P / 256π

Equating the 2 intensities,
P / 256π = 0.5P / 4πr_n²
Distance r_n = √32 = √(16 x 2) = √16 √2 = 4√2 m










Question 411: [Current Electricity]
Protons in a parallel beam each move at a uniform velocity v, thus forming a current I. The charge on each proton is e.
Which expression represents the number of protons presents in unit length of the beam? (You may wish to consider the units of the quantities involved.)
A I / e                          B I / ev                                    C Iv / e                                    D I / v

Reference: Past Exam Paper – N97 / I / 14



Solution 411:
Answer: B.
We need the number of protons in unit length of the beam. This has unit of m^-1 (number of protons has no units).
Total charge, Q = It    where t is the time
Total charge, Q = ne   where n is the number of protons in the beam
Thus,
ne = It

Let the length of the beam = L
Velocity v of a proton = L / t

Now, again consider the equation
ne = It
Number of proton, n = It / e

The number of proton per unit length = n / L. So, divide by L on both sides.
Number of proton per unit length, (n / L) = It / eL = (I/e) (t/L) = (I/e) (1/v) = I / ev










Question 412: [Nuclear Physics]

α-particle A approaches and passes by stationary gold nucleus N. Path is illustrated in Fig.

(a) On Fig, mark angle of deviation D of this α-particle as a result of passing nucleus N.

(b) Second α-particle B has same initial direction and energy as α-particle A.

On Fig, complete path of α-particle B as it approaches and passes by nucleus N.

(c) State what can be inferred about atoms from observation that very few α-particles experience large deviations.

(d) Nucleus N could be one of several different isotopes of gold.

Suggest, with explanation, whether different isotopes of gold would give rise to different deviations of a particular α-particle.

Reference: Past Exam Paper – November 2009 Paper 21 Q7



Solution 412:

(a) The deviation should be shown correctly

(b) There is a smaller deviation (not zero deviation), following an acceptable path with respect to the position of N

(c) The nucleus is (very) small in comparison to the atom

(d) The deviation depends on the charge on the nucleus / N / electrostatic repulsion. Since the different isotopes have the same charge, there is no change in the deviation.

Question 413: [Current of Electricity]

(a) Define resistance of a resistor.

(b) In circuit of Fig, battery has an e.m.f. of 3.00 V and an internal resistance r. R is a variable resistor. Resistance of ammeter is negligible and voltmeter has infinite resistance.

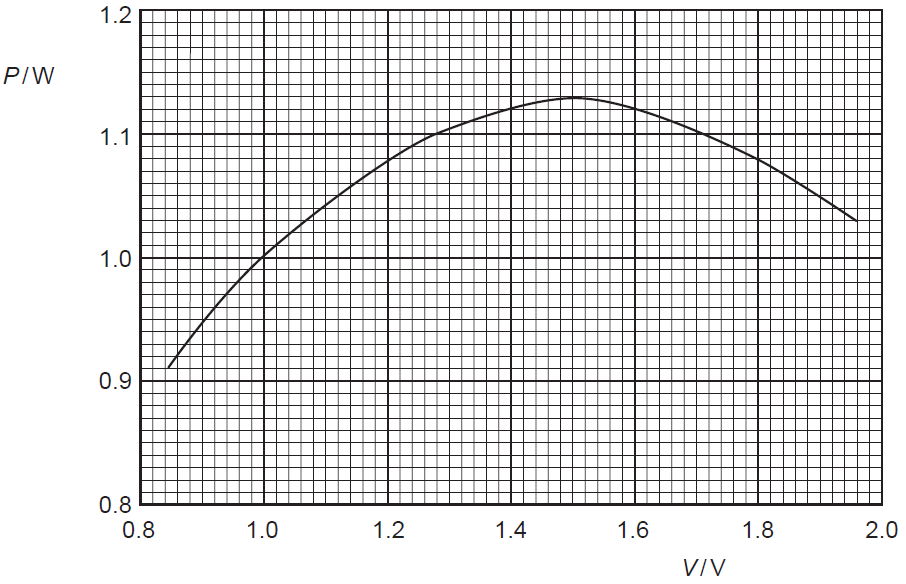
Resistance of R is varied. Fig shows variation of the power P dissipated in R with potential difference V across R.

(i) Use Fig to determine

1. maximum power dissipation in R

2. potential difference across R when the maximum power is dissipated

(ii) Hence calculate resistance of R when maximum power is dissipated.

(iii) Use answers in (i) and (ii) to determine internal resistance r of the battery.

(c) By reference to Fig, it can be seen that there are two values of potential difference V for which power dissipation is 1.05W.

State, with reason, which value of V will result in less power being dissipated in internal resistance.

Reference: Past Exam Paper – June 2005 Paper 2 Q7



Solution 413:

(a) The resistance of a resistor is the ratio of potential difference across it to the current flowing through it.

(b)

(i) Use Fig to determine

1. Maximum power dissipation in R = 1.13W

2. p.d. across R for maximum power dissipated = 1.50V

(ii)

Power dissipated = V² / R      or power = VI and V = IR

Resistance R = 1.50² / 1.13 = 1.99 Ω

(iii)

EITHER

e.m.f. E = IR + Ir

{For resistor R, V = IR. I = V / R = 1.5 / 2.0 = 0.75A}

Current I = 1.5 / 2.0 (= 0.75A)

{E = IR + Ir}

3.0 = 1.5 + 0.75r

Internal resistance, r = 2.0 Ω

OR

The voltage is divided between resistor R and internal resistance, r.

The p.d. across R is equal to the p.d. across r = 1.5.

So, resistance R = internal resistance r = 1.99 Ω

(c) A larger p.d. across resistance R means a smaller p.d. across internal resistance r {since the sum of p.d. of the 2 is constant and equal to the e.m.f.}. So, there is a smaller power dissipation {in the internal resistance r} at a larger value of V {as stated before, a greater value of V means a smaller p.d. across r} since power is given by VI {where V here is the p.d. across internal resistance r, not V from the graph} and current I is the same for R and r {the battery and the variable resistor are connected in series, not parallel. So, the same current flows through them}.