Complex Analysis: #26 Gamma Function I

• Complex Analysis: #26 The Gamma Function I

In the analysis lecture, we defined the gamma function for real numbers x > 1 using the improper integral

We can do the same thing here in the realms of complex analysis. Just substitute the complex number z for the real number x in the formula. Using the same argument as in the real case, we find that the integral converges if Re(z) > 1. But by doing this, we miss out on the fact that the gamma function can be defined everywhere in  ℂ (with isolated poles). Of course it is again possible to look at the functional equation for the gamma function, then bring in analytic continuation. But this is a rather complicated manipulation! In reality, an infinite product representation is simpler.

Let’s begin by looking at the following function

We will be using the second expression here, although the first expression shows that we can apply theorem 48 and conclude that the product does converge to an entire function, whose zeros are simply the set of negative real integers. Taking a look at the product representation of the sine function which we obtained in the last section, we see that

The function zG(z) obviously has as it’s zeros the negative real integers, and also 0. But then, if we take the function G(z − 1), we see that it also has the negative real integers and 0 as it’s set of zeros. Since all of these zeros are simple zeros for both functions, we have

being an entire function which is never zero. It is now an exercise to show that there must exist an entire function γ : ℂ → ℂ such that

ze^γ(z)G(z) = G(z − 1) 

for all z ∈ ℂ.

Let’s take the logarithmic derivative on both sides of the equation. We get

so the sum is certainly absolutely convergent and thus the third equation is valid. But this means that γ '(z) = 0, that is, γ(z) is a constant, which we simply denote by γ. In fact it is Euler’s constant.

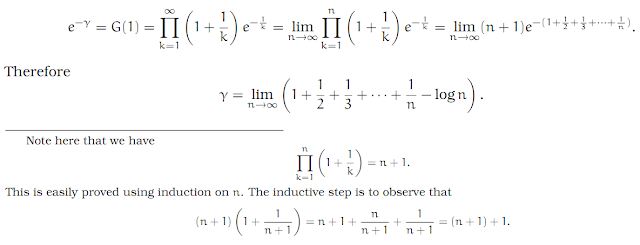
To see this, consider the case z = 1. From the formula defining G, we certainly have G(0) = 1. Therefore G(0) = 1 = e^γG(1), or

So G is an entire function satisfying the functional equation G(z−1) = ze^γG(z). In order to improve the appearance of things, let us define H(z) = G(z)e^z·γ. Then we have the functional equation

H(z − 1) = zH(z). 

This is beginning to look like the functional equation for the gamma function, but unfortunately (or fortunately?) it is going in the “wrong” direction. To fix this up, we take

From the construction, we see that it has simple poles at the negative integers, and at 0, but it has no zeros. Also, looking at the equation for the sine function, we can express this in terms of the gamma function: