• Physics 9702 Doubts | Help Page 94

Question 483: [Forces]
Car of mass 750 kg has horizontal driving force of 2.0 kN acting on it. It has forward horizontal acceleration of 2.0 m s^–2.

What is the resistive force acting horizontally?
A 0.50 kN                   B 1.5 kN                     C 2.0 kN                     D 3.5 kN

Reference: Past Exam Paper – June 2008 Paper 1 Q11 & June 2013 Paper 11 Q12



Solution 483:
Answer: A.
Resultant force = ma = 750 (2.0) = 1500N = 1.5kN

Resultant force = Driving force – Resistive force
Resistive force = Driving force – Resultant force = 2.0 – 1.5 = 0.5kN










Question 484: [Kinematics]
Aeroplane travels at average speed of 600 km h^–1 on an outward flight and at 400 km h^–1 on return flight over the same distance.
What is the average speed of whole flight?
A 111 m s^–1                 B 167 m s^–1                 C 480 km h^–1               D 500 km h^–1

Reference: Past Exam Paper – November 2013 Paper 11 & 12 Q8



Solution 484:
Answer: C.

Some may give the average as (600 + 400) / 2 = 500 km h^-1. [Choice D] But this is wrong. Averages are not that simple unless the 2 times are the same and not the 2 distances.

Average speed = Total distance / Total time

Let distance travelled for outward flight, which is equal to the distance travelled for inward flight = d. So, total distance travelled for the outward and return flights = 2d.

For each flight speed, v = d / (time, t). The time will be different since the speed is different for the flights.

let time for outward flight = t_out.        t_out = (d/600) h.           [h: hour]

let time for inward flight = t_in.                       t_in = (d/400) h.

Total time, t = t_out + t_in = d/600 + d/400 = (d/240) h

Average speed = total distance / total time = 2d / (d/240) = 480 km h^-1

Question 485: [Current of Electricity]
Diagram shows part of a current-carrying circuit. Ammeter has negligible internal resistance.

What is the reading on ammeter?
A 0.7 A                       B 1.3 A                       C 1.5 A                       D 1.7 A

Reference: Past Exam Paper – June 2011 Paper 13 Q37



Solution 485:
Answer: C.
The equivalent resistance in the circuit = R_eq
1 / R_eq = 1/1 + 1/2 + 1/5
Equivalent resistance, R_eq = [1/1 + 1/2 + 1/5]^-1 = [17/10]^-1 = (10/17) Ω

p.d. across the connection = IR_eq = 5 (10/17) = (50/17) V  

From Kirchhoff’s law, the p.d. across each loop is the same.

Let the current across the 2.0 Ω resistor be I₂.
Ohm’s law: V = IR
Current I₂ = (50/17) / 2 = 50/34 = 1.47 = 1.5A










Question 486: [Measurements]
In circuit shown, an analogue ammeter is to be recalibrated as a thermometer. Graph shows how resistance R of the thermistor changes with temperature T.

Which diagram could represent temperature scale on the ammeter?

Reference: Past Exam Paper – November 2014 Paper 13 Q3



Solution 486:

Answer: A.

The graph shown is that of resistance R of the thermistor (not current) against temperature T.

Ohm’s law: V = IR    

Current I = V / R. So, the current is inversely proportional to the resistance R. The ammeter measures the current, not the resistance in a circuit.

The resistance falls rapidly with temperature at low temperatures, so the current will rise rapidly at low temperatures. Therefore, we need a larger region of the scale to represent a fixed amount of change in current at low temperatures. If the values on the scales are close to each other, the change in readings may not be noticeable.

The resistance curve becomes flatter at higher temperatures, so the current will change more slowly with temperature. Therefore, to represent this region of large temperature, a relatively smaller region of the scale is required. So, the values of the scale can be closer to each other.

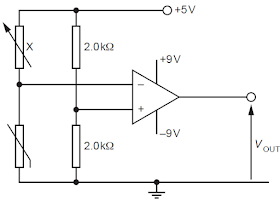
Question 487: [Operational Amplifier]

(a) An operational amplifier (op-amp) may be used as comparator.

State function of a comparator.

(b) Variation with temperature θ of resistance R of a thermistor is shown in Fig.

Thermistor is connected into the circuit of Fig.

Op-amp may be considered to be ideal.

(i) Temperature of thermistor is 10 °C.

Determine resistance of the variable resistor X such that output potential V_OUT is zero.

(ii) Resistance of resistor X is now held constant at the value calculated in (i). Describe change in the output potential V_OUT as temperature of the thermistor is changed from 5 °C to 20 °C.

Reference: Past Exam Paper – June 2011 Paper 41 Q9



Solution 487:

(a) A comparator is used to compare two potentials / voltages. The output depends upon which is greater.

(b)

(i)

Resistance of thermistor = 2.5 kΩ

{V_OUT = 10⁵ (V⁺ – V^-) where the open-loop gain = 10⁵.

V+ may be obtained by considering the two 2.0 kΩ. The total potential difference across them is 5V. Since both have the same resistance, the p.d. across each of them is 5 / 2 = 2.5V. So, V⁺ = 2.5V.

V_OUT is said to be zero. Therefore, V^- must be equal to V⁺ = 2.5V. This is half the total potential of 5V across both the thermistor and resistor X. So, for this to occur, both the thermistor and the resistor X should have the same p.d. across them. So, resistance of resistor X= 2.5 kΩ.}

Resistance of resistor X= 2.5 kΩ

(ii) (At 5 °C or) For temperatures less than 10 °C, V^– is greater than V⁺ so V_OUT is –9 V. (at 10 °C or) For temperatures greater than 10 °C, V^– is less than V⁺ and V_OUT is +9 V. V_OUT switches between negative and positive at 10 °C.

Question 488: [Matter > Deformation]

Tension in a spring of natural length l₀ is first increased from zero to T₁, causing length to increase to l₁. Tension is then reduced to T₂, causing length to decrease to l₂ (as shown).

Which area of the graph represents the work done by the spring during this reduction in length?

A MLP                        B MNQP                     C MNSR                     D MPLU

Reference: Past Exam Paper – June 2002 Paper 1 Q24

Solution 488:

Answer: B.

The work done by the spring is given by the area under the tension-length graph.

The reduction is from l₁ to l₂, corresponding to points P and Q respectively. The tension changes from T₁ (point M) to T₂ (point N). Thus, we need to consider the area of the trapezium MNQP formed.